Question 707 continuously compounding rate, continuously compounding rate conversion
Convert a 10% effective annual rate ##(r_\text{eff annual})## into a continuously compounded annual rate ##(r_\text{cc annual})##. The equivalent continuously compounded annual rate is:
Question 708 continuously compounding rate, continuously compounding rate conversion
Convert a 10% continuously compounded annual rate ##(r_\text{cc annual})## into an effective annual rate ##(r_\text{eff annual})##. The equivalent effective annual rate is:
Which of the following interest rate quotes is NOT equivalent to a 10% effective annual rate of return? Assume that each year has 12 months, each month has 30 days, each day has 24 hours, each hour has 60 minutes and each minute has 60 seconds. APR stands for Annualised Percentage Rate.
Since the assumptions state that there are 30 days per month and therefore 360 days per year, then the annualised percentage rate compounding per day should be:
###\begin{aligned} r_\text{APR comp daily} &= r_\text{eff daily} \times 360 \\ &= ((1 + r_\text{eff annual})^{1/360}-1) \times 360 \\ &= ((1 + 0.1)^{1/360}-1) \times 360 \\ &= 0.00026478555 \times 360 \\ &= 0.095322798 \\ \end{aligned}###Commentary
Notice that the APR's get smaller as the compounding period becomes shorter. The continuously compounded return is the limit when the compounding period is infinitely small. The APR compounding per second is nearly equal to the continuously compounded rate.
| Different Return Quotations Equivalent to an Effective Annual Rate of 10% | ||||
| Quote type | Return (%pa) | Symbol | Formula | Spreadsheet formula |
| Effective annual rate | 10 | ##r_\text{eff annual}## | ##=r_\text{eff annual}## | =0.1 |
| APR compounding per annum | 10 | ##r_\text{apr comp annually}## | ##=r_\text{eff annual}## | =0.1 |
| APR compounding semi-annually | 9.761769634 | ##r_\text{apr comp 6mth}## | ##=2 \times ((1+r_\text{eff annual})^{1/2}-1)## | =2 * ((1+0.1)^(1/2)-1) |
| APR compounding quarterly | 9.645475634 | ##r_\text{apr comp quarterly}## | ##=4 \times ((1+r_\text{eff annual})^{1/4}-1)## | =4 * ((1+0.1)^(1/4)-1) |
| APR compounding monthly | 9.568968515 | ##r_\text{apr comp monthly}## | ##=12 \times ((1+r_\text{eff annual})^{1/12}-1)## | =12 * ((1+0.1)^(1/12)-1) |
| APR compounding daily | 9.532279763 | ##r_\text{apr comp daily}## | ##=360\times ((1+r_\text{eff annual})^{1/360}-1)## | =360 * ((1+0.1)^(1/360)-1) |
| APR compounding hourly | 9.531070550 | ##r_\text{apr comp hourly}## | ##=360 \times 24 \times ((1+r_\text{eff annual})^{1/(360 \times 24)}-1)## | =360*24 * ((1+0.1)^(1/(360*24))-1) |
| APR compounding per minute | 9.531018861 | ##r_\text{apr comp per minute}## | ##=360 \times 24 \times 60 \times ((1+r_\text{eff annual})^{1/(360 \times 24 \times 60)}-1)## | =360*24*60 * ((1+0.1)^(1/(360*24*60))-1) |
| APR compounding per second | 9.531018227 | ##r_\text{apr comp per second}## | ##=360 \times 24 \times 60 \times 60 \times ((1+r_\text{eff annual})^{1/(360 \times 24 \times 60 \times 60)}-1)## | =360*24*60*60 * ((1+0.1)^(1/(360*24*60*60))-1) |
| Continuously compounded annual rate | 9.531017980 | ##r_\text{cc annual}## | ##=\ln(1+r_\text{eff annual}) = log_e(1+r_\text{eff annual})## | =ln(1+0.1) |
Question 710 continuously compounding rate, continuously compounding rate conversion
A continuously compounded monthly return of 1% ##(r_\text{cc monthly})## is equivalent to a continuously compounded annual return ##(r_\text{cc annual})## of:
Converting continuously compounding rates to different time periods is surprisingly easy. To compound up from monthly to annual, just multiply by the number of months in a year.
###\begin{aligned} r_\text{cc annual} &= r_\text{cc monthly} \times 12 \\ &= 0.01 \times 12 \\ &= 0.12 \\ \end{aligned}###Question 711 continuously compounding rate, continuously compounding rate conversion
A continuously compounded semi-annual return of 5% ##(r_\text{cc 6mth})## is equivalent to a continuously compounded annual return ##(r_\text{cc annual})## of:
To compound up from semi-annual to annual, just multiply by 2 which is the number of 6 month periods in a year.
###\begin{aligned} r_\text{cc annual} &= r_\text{cc 6mth} \times 2 \\ &= 0.05 \times 2 \\ &= 0.1 \\ \end{aligned}###An effective monthly return of 1% ##(r_\text{eff monthly})## is equivalent to an effective annual return ##(r_\text{eff annual})## of:
Converting effective rates to different time periods requires powers to take compounding into account. To compound up from monthly to annual, add one, raise to the power of the number of months in a year, then subtract one.
###\begin{aligned} r_\text{eff annual} &= (1+r_\text{eff monthly})^{12}-1 \\ &= (1+0.01)^{12}-1 \\ &= 0.12682503 \\ \end{aligned}###An effective semi-annual return of 5% ##(r_\text{eff 6mth})## is equivalent to an effective annual return ##(r_\text{eff annual})## of:
Converting effective rates to different time periods is requires powers to take compounding into effect. To compound up from semi-annual to annual, add one, raise to the power of 2, the number of semi-annual periods in a year, then subtract one.
###\begin{aligned} r_\text{eff annual} &= (1+r_\text{eff 6mth})^{2}-1 \\ &= (1+0.05)^{2}-1 \\ &= 0.1025 \\ \end{aligned}###Question 691 continuously compounding rate, effective rate, continuously compounding rate conversion, no explanation
A bank quotes an interest rate of 6% pa with quarterly compounding. Note that another way of stating this rate is that it is an annual percentage rate (APR) compounding discretely every 3 months.
Which of the following statements about this rate is NOT correct? All percentages are given to 6 decimal places. The equivalent:
No explanation provided.
Which of the following quantities is commonly assumed to be normally distributed?
No explanation provided.
If a variable, say X, is normally distributed with mean ##\mu## and variance ##\sigma^2## then mathematicians write ##X \sim \mathcal{N}(\mu, \sigma^2)##.
If a variable, say Y, is log-normally distributed and the underlying normal distribution has mean ##\mu## and variance ##\sigma^2## then mathematicians write ## Y \sim \mathbf{ln} \mathcal{N}(\mu, \sigma^2)##.
The below three graphs show probability density functions (PDF) of three different random variables Red, Green and Blue.
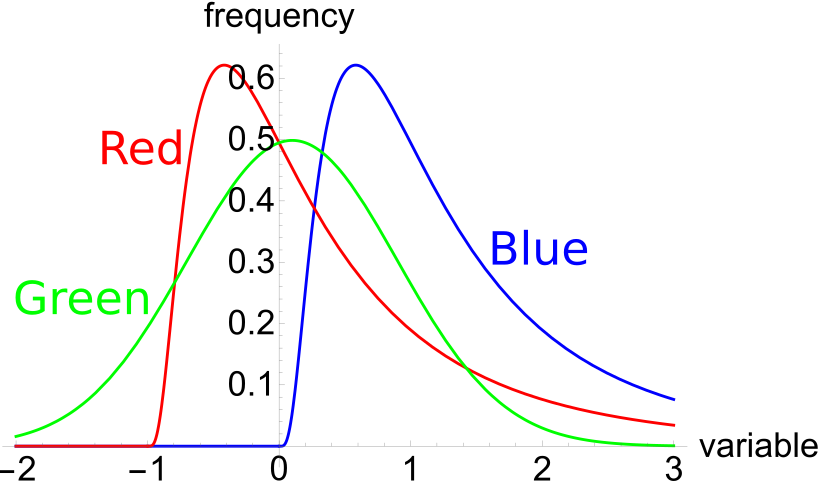
Select the most correct statement:
Variables Red and Blue are log-normally distributed since they are not symmetric, they're skewed. They have a fat right tail which stretches to positive infinity, but the left tail has a minimum point.
Variable Green has the symmetric bell-shape typical of a normal distribution which has left and right tails that continue to negative and positive infinity respectively.
The below three graphs show probability density functions (PDF) of three different random variables Red, Green and Blue.

Which of the below statements is NOT correct?
The variable Green is always between ##-\infty < Green < \infty## if it is normally distributed, there is no minimum or maximum value. This is because a normal distribution has left and right tails that stretch to negative and positive infinity respectively.
The below three graphs show probability density functions (PDF) of three different random variables Red, Green and Blue. Let ##P_1## be the unknown price of a stock in one year. ##P_1## is a random variable. Let ##P_0 = 1##, so the share price now is $1. This one dollar is a constant, it is not a variable.

Which of the below statements is NOT correct? Financial practitioners commonly assume that the shape of the PDF represented in the colour:
The shape of the PDF represented in Red is commonly assumed to be the effective rate of return which is equal to the net discrete return. They are synonyms.
###r_\text{eff} = \text{NDR} = \text{GDR} - 1 = P_1/P_0-1 = (P_1-P_0)/P_0###The net discrete return equals the gross discrete return (Blue) minus one, which shifts the graph to the left by one unit. You can see that the Red PDF is the same as the Blue one, but shifted one unit to the left.
Note that the price and gross discrete return variables have the exact same PDF if the price now ##(P_0)## is one dollar, as stated in the assumptions. If the price is some other number, then the gross discrete return PDF will be a scaled version of stock price PDF since the gross discrete return is equal to the unknown future price divided by the known current price which is just a constant. For example, if the current stock price was $2 then the PDF of the price next year ##(P_1)## would look the same as the gross discrete return PDF but stretched along the x-axis to be twice as wide.
The mean of a log-normally distributed variable is always higher than the median because the median is the middle value (the 50th percentile) which is less influenced by outliers.
The mean is more heavily influenced by outliers, especially in the log-normal distribution where the mean is pulled higher by the very large returns in the far right tail.
The symbol ##\text{GDR}_{0\rightarrow 1}## represents a stock's gross discrete return per annum over the first year. ##\text{GDR}_{0\rightarrow 1} = P_1/P_0##. The subscript indicates the time period that the return is mentioned over. So for example, ##\text{AAGDR}_{1 \rightarrow 3}## is the arithmetic average GDR measured over the two year period from years 1 to 3, but it is expressed as a per annum rate.
Which of the below statements about the arithmetic and geometric average GDR is NOT correct?
Statement b is false. The geometric average return is the product of the returns raised to the power of 1 on the number of returns:
###\text{GAGDR}_{0\rightarrow T} = \left( \text{GDR}_{0 \rightarrow 1} . \text{GDR}_{1\rightarrow 2} ... \text{GDR}_{T-1 \rightarrow T} \right)^{\mathbf{1/T}}###Statement e is true. The arithmetic and geometric averages of returns will be equal if the variance of the stock's returns is zero. But this would be very unusual because the stock return would then be constant and therefore risk free.
Question 811 log-normal distribution, mean and median returns, return distribution, arithmetic and geometric averages
Which of the following statements about probability distributions is NOT correct?
A stocks’ future annual net discrete returns ##(P_t/P_0-1)## must be log-normally distributed when future stock prices ##(P_t)## are also log-normally distributed. This is because net discrete returns are 'linear transformations' of stock prices. The shape of the net discrete return's distribution (given by its probability density function or PDF) is the same as the future stock price's log-normal distribution, but it's squashed flatter when it's divided by the original price ##(P_t/\color{red}{P_0}-1)##, and shifted to the left by one unit due to the minus one ##(P_t/P_0\color{red}{-1})##.
Question 721 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
Fred owns some Commonwealth Bank (CBA) shares. He has calculated CBA’s monthly returns for each month in the past 20 years using this formula:
###r_\text{t monthly}=\ln \left( \dfrac{P_t}{P_{t-1}} \right)###He then took the arithmetic average and found it to be 1% per month using this formula:
###\bar{r}_\text{monthly}= \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( r_\text{t monthly} \right)} }{T} =0.01=1\% \text{ per month}###He also found the standard deviation of these monthly returns which was 5% per month:
###\sigma_\text{monthly} = \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( \left( r_\text{t monthly} - \bar{r}_\text{monthly} \right)^2 \right)} }{T} =0.05=5\%\text{ per month}###Which of the below statements about Fred’s CBA shares is NOT correct? Assume that the past historical average return is the true population average of future expected returns.
Over the next 10 years the expected mean gross discrete 10 year return is:
###\text{MeanGDR} = \text{AAGDR} = e^{(AALGDR + SDLGDR^2/2).t} = e^{(0.01 + 0.05^2/2)×12×10} = 3.857425531###The expected median gross discrete 10 year return is:
###\text{MedianGDR} = \text{GAGDR} = e^{AALGDR.t} = e^{0.01×12×10}=3.320116923###Note that the gross discrete return is log-normally distributed so the mean will always be greater than the median.
Question 722 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
Here is a table of stock prices and returns. Which of the statements below the table is NOT correct?
| Price and Return Population Statistics | ||||
| Time | Prices | LGDR | GDR | NDR |
| 0 | 100 | |||
| 1 | 50 | -0.6931 | 0.5 | -0.5 |
| 2 | 100 | 0.6931 | 2 | 1 |
| Arithmetic average | 0 | 1.25 | 0.25 | |
| Arithmetic standard deviation | 0.9802 | 1.0607 | 1.0607 | |
Statement e is false and it can be verified using the actual data in the table, which is done below. The logarithm of the arithmetic average of the gross discrete returns (LAAGDR) is only asymptotically equal to the arithmetic average of the logarithms of the gross discrete returns (AALGDR) plus half the variance of the LGDR's. It's only true if the LGDR's are normally distributed and there are lots of observations.
###\text{LAAGDR} \approx \text{AALGDR} + \text{SDLGDR}^2/2###Note that ##\approx## means 'approximately equal to'. The equation will only be equal at the limit as the time period that the averages are measured over reaches infinity.
###\lim_{T \to \infty} (LAAGDR) = \lim_{T \to \infty} (\text{AALGDR} + \text{SDLGDR}^2/2)###Let's check that the two sides of the equation are approximately equal, but not exactly equal, using the table data:
###\text{LAAGDR} \approx \text{AALGDR} + \text{SDLGDR}^2/2### ###\ln(1.25) \approx 0 + 0.98019142^2/2### ###0.223143551 \approx 0.48038761###The figures are quite different, but if more time periods were added, then these values would converge to be approximately equal. One reason why the figures are different is because the two gross discrete return data points are not log-normally distributed. Remember that the log-normal distribution is supposed to be skewed but with only two return observations it's impossible to create skew.
Statement d is true. The logarithm of the geometric average of the gross discrete returns (LGAGDR) is equal to the arithmetic average of the logarithms of the gross discrete returns (AALGDR). This is always true, regardless of the distribution of returns.
###\text{LGAGDR} = \text{AALGDR}### Where: ###\text{LGAGDR} = \ln \left( \left( \displaystyle\prod\limits_{t=1}^T{\left( \text{GDR}_t \right)} \right)^{1/T} \right) ### ###\text{AALGDR} = \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( \text{LGDR}_t \right)} }{T} ###Let's check the equation using the table data:
###\ln(\text{GAGDR}) = \text{AALGDR}### ###\ln(1) = 0### ###0 = 0###Statement a is true. The geometric average of the gross discrete returns (GAGDR) is equal to:
###\text{GAGDR} = \left( \displaystyle\prod\limits_{t=1}^T{\left( \text{GDR}_t \right)} \right)^{1/T} = (0.5 \times 2)^{1/2} = 1 = 100\%###Interestingly, another way to calculate the GAGDR is to find the natural exponent of the arithmetic average of the logarithms of the gross discrete returns (AALGDR).
This means that statement b is also true:
###\text{GAGDR} = \exp \left( \text{AALGDR} \right) = \exp \left( 0 \right) = e^0 = 1###Statement c is true. The geometric average gross discrete return is also equal to the last price divided by the first price raised to the power of the inverse number of time periods between them.
###\text{GAGDR} = \left( P_T/P_0 \right)^{1/T} = (100/100)^{1/2} = 1 = 100\%###The mean of a normally distributed variable is always (asymptotically) equal to the median because the normal distribution is symmetric. Both the left and right tails are equal so outliers on each side tend to cancel each other out and do not affect either the median or mean (or mode). All three would be expected to be equal.
The mean of a log-normally distributed variable is always higher than the median because the median is the middle value (the 50th percentile) which is less influenced by outliers.
The mean is more heavily influenced by outliers, especially in the log-normal distribution where the mean is pulled higher by the very large returns in the fat right tail.
Question 719 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
A stock has an arithmetic average continuously compounded return (AALGDR) of 10% pa, a standard deviation of continuously compounded returns (SDLGDR) of 80% pa and current stock price of $1. Assume that stock prices are log-normally distributed. The graph below summarises this information and provides some helpful formulas.
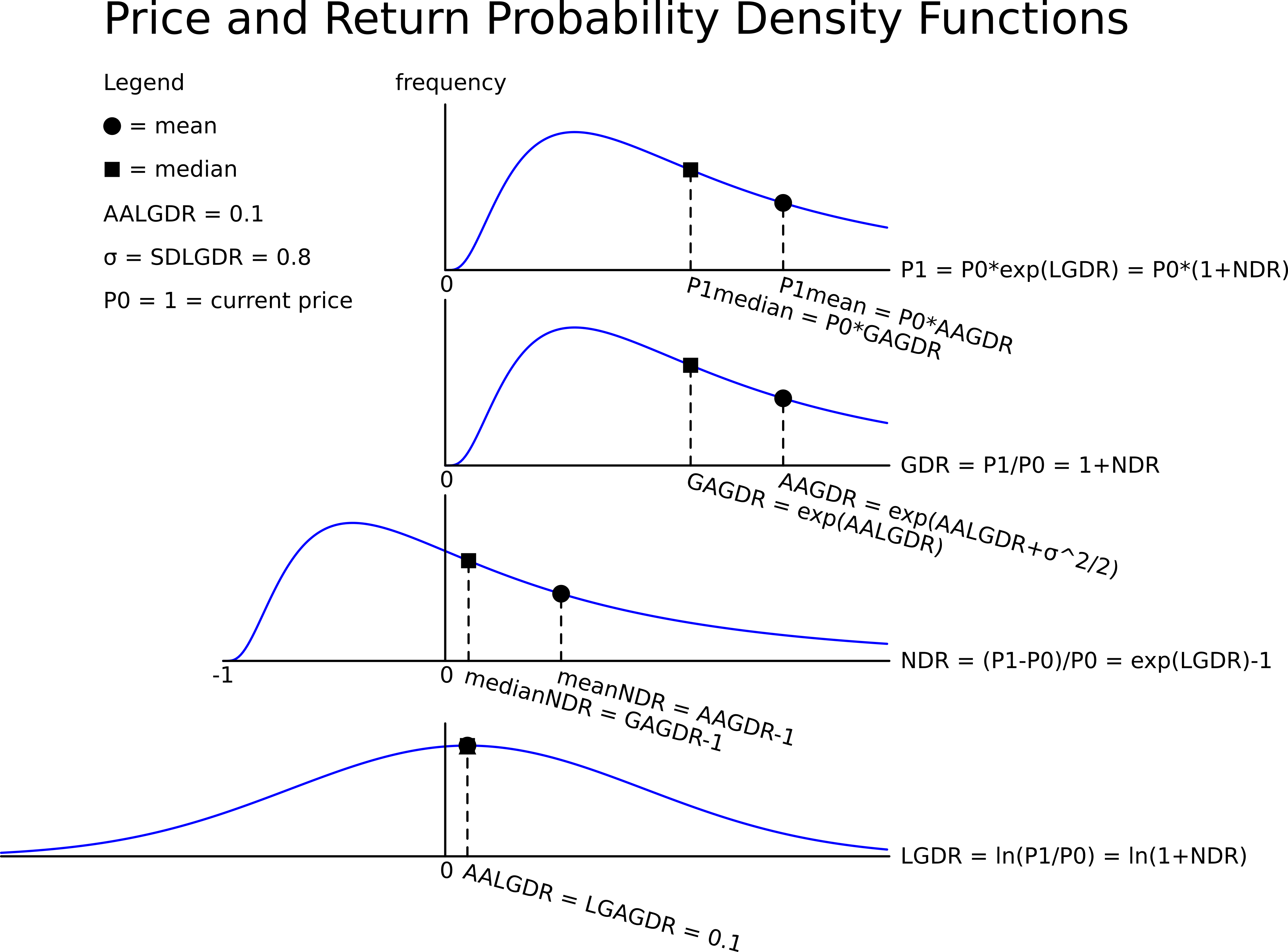
In one year, what do you expect the median and mean prices to be? The answer options are given in the same order.
The median price can be found by compounding up at the arithmetic average continuously compounded return ##(\text{AALGDR})##.
###\text{MedianP}_t = P_0.e^{\text{AALGDR}.t}### ###\begin{aligned} \text{MedianP}_{1} &= 1\times e^{0.1 \times 1} \\ &= 1.105170918 \\ \end{aligned}###The mean price can be found by compounding up at the arithmetic average continuously compounded return ##(\text{AALGDR})## plus half the variance ##(\text{SDLGDR}^2/2)##.
###\text{MeanP}_t = P_0.e^{(\text{AALGDR}+\text{SDLGDR}^2/2).t}### ###\begin{aligned} \text{MeanP}_{1} &= 1 \times e^{(0.1+0.8^2/2) \times 1} \\ &= 1.521961556 \\ \end{aligned}###
Question 720 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
A stock has an arithmetic average continuously compounded return (AALGDR) of 10% pa, a standard deviation of continuously compounded returns (SDLGDR) of 80% pa and current stock price of $1. Assume that stock prices are log-normally distributed.
In 5 years, what do you expect the median and mean prices to be? The answer options are given in the same order.
The median price can be found by compounding up at the arithmetic average continuously compounded return ##(\text{AALGDR})##.
###\text{MedianP}_t = P_0.e^{\text{AALGDR}.t}### ###\begin{aligned} \text{MedianP}_{5} &= 1\times e^{0.1 \times 5} \\ &= 1.648721271 \\ \end{aligned}###The mean price can be found by compounding up at the arithmetic average continuously compounded return ##(\text{AALGDR})## plus half the variance ##(\text{SDLGDR}^2/2)##.
###\text{MeanP}_t = P_0.e^{(\text{AALGDR}+\text{SDLGDR}^2/2).t}### ###\begin{aligned} \text{MeanP}_{5} &= 1 \times e^{(0.1+0.8^2/2) \times 5} \\ &= 8.166169913 \\ \end{aligned}###Question 723 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
Here is a table of stock prices and returns. Which of the statements below the table is NOT correct?
| Price and Return Population Statistics | ||||
| Time | Prices | LGDR | GDR | NDR |
| 0 | 100 | |||
| 1 | 99 | -0.010050 | 0.990000 | -0.010000 |
| 2 | 180.40 | 0.600057 | 1.822222 | 0.822222 |
| 3 | 112.73 | 0.470181 | 0.624889 | 0.375111 |
| Arithmetic average | 0.0399 | 1.1457 | 0.1457 | |
| Arithmetic standard deviation | 0.4384 | 0.5011 | 0.5011 | |
Statement b is false since it mixes the two average returns up.
###\exp \left( \text{GAGDR} \right) \neq \text{AALGDR}###The GAGDR equals the natural exponential of the arithmetic average of the logarithms of the gross discrete returns ##(\exp(\text{AALGDR})##.
###\begin{aligned} \text{GAGDR} &= \exp \left( \text{AALGDR} \right) \\ &= \exp \left( 0.0399 \right) \\ &= e^{0.0399} = 1.04075 \\ \end{aligned}###Statement a is true. The geometric average of the gross discrete returns (GAGDR) is equal to:
###\begin{aligned} \text{GAGDR} &= \left( \displaystyle\prod\limits_{t=1}^T{\left( \text{GDR}_t \right)} \right)^{1/T} \\ &= (0.990000 \times 1.822222 \times 0.624889)^{1/3} \\ &= 1.04075 = 104.075\% \\ \end{aligned}###Statement c is true. The geometric average gross discrete return is also equal to the last price divided by the first price raised to the power of the inverse number of time periods between them.
###\begin{aligned} \text{GAGDR} &= \left( P_T/P_0 \right)^{1/T} \\ &= (112.73/100)^{1/3} = 1.04075\\ \end{aligned}###Statement d is true. The logarithm of the geometric average of the gross discrete returns (LGAGDR) is equal to the arithmetic average of the logarithm of the gross discrete returns (AALGDR). This is always true, regardless of the distribution of returns.
###\text{LGAGDR} = \text{AALGDR}### Where: ###\text{LGAGDR} = \ln \left( \left( \displaystyle\prod\limits_{t=1}^T{\left( \text{GDR}_t \right)} \right)^{1/T} \right) ### ###\text{AALGDR} = \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( \text{LGDR}_t \right)} }{T} ###Let's check the equation using the table data:
###\ln(\text{GAGDR}) = \text{AALGDR}### ###\ln(1.04075) = 0.0399### ###0.0399 = 0.0399###Note that due to rounding in the GAGDR and AALGDR figures given in the table, the numbers aren't exactly equal after the 4th decimal place.
Statement e is true. The logarithm of the arithmetic average of the gross discrete returns (LAAGDR) is only asymptotically equal to the arithmetic average of the logarithm of the gross discrete returns (AALGDR) plus half the variance of the LGDR's. It's only true if the LGDR's are normally distributed and there are lots of observations.
###\text{LAAGDR} \approx \text{AALGDR} + \text{SDLGDR}^2/2###Note that ##\approx## means 'approximately equal to'. The equation will only be equal at the limit as the time period that the averages are measured over reaches infinity.
###\lim_{T \to \infty} (\text{AALGDR}) = \text{LGAGDR}###Let's check that the two sides of the equation are approximately equal using the table data:
###\text{LAAGDR} \approx \text{AALGDR} + \text{SDLGDR}^2/2### ###\ln(1.1457) \approx 0.0399 + 0.4384^2/2### ###0.136015804 = 0.13599728###So they are approximately equal, but the prices in the table were deliberately chosen to give this result. In actual stock price data with only 3 return observations such as here, the result would rarely hold.
Question 779 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
Fred owns some BHP shares. He has calculated BHP’s monthly returns for each month in the past 30 years using this formula:
###r_\text{t monthly}=\ln \left( \dfrac{P_t}{P_{t-1}} \right)###He then took the arithmetic average and found it to be 0.8% per month using this formula:
###\bar{r}_\text{monthly}= \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( r_\text{t monthly} \right)} }{T} =0.008=0.8\% \text{ per month}###He also found the standard deviation of these monthly returns which was 15% per month:
###\sigma_\text{monthly} = \dfrac{ \displaystyle\sum\limits_{t=1}^T{\left( \left( r_\text{t monthly} - \bar{r}_\text{monthly} \right)^2 \right)} }{T} =0.15=15\%\text{ per month}###Assume that the past historical average return is the true population average of future expected returns and the stock's returns calculated above ##(r_\text{t monthly})## are normally distributed. Which of the below statements about Fred’s BHP shares is NOT correct?
The mean price in 10 years will be higher than the median price since the price must be log-normally distributed due to the continuously compounded return being normally distributed.
###\begin{aligned} \text{MeanPrice}_\text{10 years} &= \text{P}_\text{0} . e^{(AALGDR \mathbf{+} SDLGDR^2/2).t} \\ &= 20 \times e^{(0.008 + 0.15^2/2)×12×10} \\ &= 201.4884931 \\ \end{aligned}###The median price in 10 years is:
###\begin{aligned} \text{MedianPrice}_\text{10 years} &= \text{P}_\text{0} .e^{ \text{AALGDR}.t} \\ &= 20 \times e^{ 0.008 \times 12 \times 10} \\ &= 52.23392947 \\ \end{aligned}###The stock price is log-normally distributed so the mean will always be greater than the median.
Question 792 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate, log-normal distribution, confidence interval
A risk manager has identified that their investment fund’s continuously compounded portfolio returns are normally distributed with a mean of 10% pa and a standard deviation of 40% pa. The fund’s portfolio is currently valued at $1 million. Assume that there is no estimation error in the above figures. To simplify your calculations, all answers below use 2.33 as an approximation for the normal inverse cumulative density function at 99%. All answers are rounded to the nearest dollar. Assume one month is 1/12 of a year. Which of the following statements is NOT correct?
The worst one percentile of portfolio values in one month is $770,503. Note that the mean return is multiplied by the time ##(1/12)## while the standard deviation of returns is multiplied by the square root of the time ##(\sqrt{1/12})##:
###\begin{aligned} \text{WorstPercentileValueInOneMonth} &= V_0.e^{\mu .t + \sigma.N[0.01].\sqrt{t}} \\ &= 1m.e^{0.1 \times 1/12 + 0.4 \times -2.33 \times \sqrt{1/12}} \\ &= 0.770502876m \\ \end{aligned}###The worst one percentile of portfolio values in one year is:
###\begin{aligned} \text{WorstPercentileValueInOneYear} &= V_0.e^{\mu .t + \sigma.N[0.01].\sqrt{t}} \\ &= 1m.e^{0.1 \times 1 + 0.4 \times -2.33 \times \sqrt{1}} \\ &= 0.435178059m \\ \end{aligned}###The medians and means are:
###\begin{aligned} \text{MedianValueInOneYear} &= V_0.e^{\mu .t} \\ &= 1m.e^{0.1 \times 1} \\ &= 1.105170918m \\ \end{aligned}### ###\begin{aligned} \text{MedianValueInOneMonth} &= V_0.e^{\mu .t} \\ &= 1m.e^{0.1 \times 1/12} \\ &= 1.008368152m \\ \end{aligned}### ###\begin{aligned} \text{MeanValueInOneYear} &= V_0.e^{(\mu + \sigma^2/2).t} \\ &= 1m.e^{(0.1 + 0.4^2/2) \times 1} \\ &= 1.197217363m \end{aligned}### ###\begin{aligned} \text{MeanValueInOneMonth} &= V_0.e^{(\mu + \sigma^2/2).t} \\ &= 1m.e^{(0.1 + 0.4^2/2) \times 1/12} \\ &= 1.015113065m \end{aligned}###The 98% confidence interval of portfolio values in two years is between 0.326917629m and 4.563304537m:
###\begin{aligned} \text{WorstOnePercentileValueInTwoYears} &= V_0.e^{\mu .t + \sigma.N[0.01].\sqrt{t}} \\ &= 1m.e^{0.1 \times 2 + 0.4 \times -2.33 \times \sqrt{2}} \\ &= 0.326917629m \\ \end{aligned}### ###\begin{aligned} \text{BestOnePercentileValueInTwoYears} &= V_0.e^{\mu .t + \sigma.N[0.99].\sqrt{t}} \\ &= 1m.e^{0.1 \times 2 + 0.4 \times 2.33 \times \sqrt{2}} \\ &= 4.563304537m \\ \end{aligned}###Question 811 log-normal distribution, mean and median returns, return distribution, arithmetic and geometric averages
Which of the following statements about probability distributions is NOT correct?
A stocks’ future annual net discrete returns ##(P_t/P_0-1)## must be log-normally distributed when future stock prices ##(P_t)## are also log-normally distributed. This is because net discrete returns are 'linear transformations' of stock prices. The shape of the net discrete return's distribution (given by its probability density function or PDF) is the same as the future stock price's log-normal distribution, but it's squashed flatter when it's divided by the original price ##(P_t/\color{red}{P_0}-1)##, and shifted to the left by one unit due to the minus one ##(P_t/P_0\color{red}{-1})##.
Question 877 arithmetic and geometric averages, utility, utility function
Gross discrete returns in different states of the world are presented in the table below. A gross discrete return is defined as ##P_1/P_0##, where ##P_0## is the price now and ##P_1## is the expected price in the future. An investor can purchase only a single asset, A, B, C or D. Assume that a portfolio of assets is not possible.
| Gross Discrete Returns | ||
| In Different States of the World | ||
| Investment | World states (probability) | |
| asset | Good (50%) | Bad (50%) |
| A | 2 | 0.5 |
| B | 1.1 | 0.9 |
| C | 1.1 | 0.95 |
| D | 1.01 | 1.01 |
Which of the following statements about the different assets is NOT correct? Asset:
Asset C's Arithmetic Average Gross Discrete Return (AAGDR = 1.025) is higher than its Geometric Average Gross Discrete Return (GAGDR = 1.022252415). An asset's AAGDR is always higher than its GAGDR when there's volatility, regardless of the distribution of the asset's returns. The only time the AAGDR and GAGDR are equal is when there's zero volatility, as is the case with asset D.
| Gross Discrete Returns | |||||
| In Different States of the World | |||||
| Investment | World states (probability) | Averages | |||
| asset | Good (50%) | Bad (50%) | AAGDR | GAGDR | AALGDR or LGAGDR |
| A | 2 | 0.5 | 1.25 | 1 | 0 |
| B | 1.1 | 0.9 | 1 | 0.994987437 | -0.005025168 |
| C | 1.1 | 0.95 | 1.025 | 1.022252415 | 0.022008443 |
| D | 1.01 | 1.01 | 1.01 | 1.01 | 0.009950331 |
We'll show the working to get the results for asset A in the above table. The other assets' results can be found similarly.
To find asset A's arithmetic average gross discrete returns (AAGDR):
###AAGDR = GDR_1.w_1+ GDR_2.w_2 + GDR_3.w_3 + ... +GDR_n.w_n### ###\begin{aligned} AAGDR_A &= 2 \times 0.5 + 0.5 \times 0.5 \\ &= 1 + 0.25 \\ &= 1.25 \\ \end{aligned}###To find asset A's geometric average gross discrete returns (GAGDR):
###GAGDR = GDR_1^{w_1}.GDR_2^{w_2}.GDR_3^{w_3}. ... .GDR_n^{w_n}### ###\begin{aligned} GAGDR_A &= 2^{0.5} \times 0.5^{0.5} \\ &= 1.414213562 \times 0.707106781 \\ &= 1 \\ \end{aligned}###To find asset A's expected log utility of wealth, note that it's the same as the arithmetic average log gross discrete return (AALGDR). This is because 'expected' value means 'arithmetic average' value in mathematics and economics, so the expected log utility of wealth is just the arithmetic average of the natural logarithms of the returns:
###AALGDR = \ln{(GDR_1)}.w_1+ \ln{(GDR_2)}.w_2 + \ln{(GDR_3)}.w_3 + ... +\ln{(GDR_n)}.w_n### ###\begin{aligned} AALGDR_A &= \ln{(2)} \times 0.5 + \ln{(0.5)} \times 0.5 \\ &= 0.693147181 \times 0.5 + -0.693147181 \times 0.5 \\ &= 0 \\ \end{aligned}###Another interesting thing is that the arithmetic average log gross discrete return (AALGDR) is also equal to the log of the geometric average gross discrete return (LGAGDR). This is true regardless of the distribution of the asset's returns.
Question 925 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate, no explanation
The arithmetic average and standard deviation of returns on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 were calculated as follows:
###\bar{r}_\text{yearly} = \dfrac{ \displaystyle\sum\limits_{t=1992}^{24}{\left( \ln \left( \dfrac{P_{t+1}}{P_t} \right) \right)} }{T} = \text{AALGDR} =0.0949=9.49\% \text{ pa}###
###\sigma_\text{yearly} = \dfrac{ \displaystyle\sum\limits_{t=1992}^{24}{\left( \left( \ln \left( \dfrac{P_{t+1}}{P_t} \right) - \bar{r}_\text{yearly} \right)^2 \right)} }{T} = \text{SDLGDR} = 0.1692=16.92\text{ pp pa}###
Assume that the log gross discrete returns are normally distributed and that the above estimates are true population statistics, not sample statistics, so there is no standard error in the sample mean or standard deviation estimates. Also assume that the standardised normal Z-statistic corresponding to a one-tail probability of 2.5% is exactly -1.96.
Which of the following statements is NOT correct? If you invested $1m today in the ASX200, then over the next 4 years:
No explanation provided.
Question 926 mean and median returns, return distribution, arithmetic and geometric averages, continuously compounding rate
The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.
The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.
Assume that the log gross discrete returns are normally distributed and that the above estimates are true population statistics, not sample statistics, so there is no standard error in the sample mean or standard deviation estimates. Also assume that the standardised normal Z-statistic corresponding to a one-tail probability of 2.5% is exactly -1.96.
If you had a $1 million fund that replicated the ASX200 accumulation index, in how many years would the median dollar value of your fund first be expected to lie outside the 95% confidence interval forecast?
The median is the 50th percentile so it will never lie outside of the 95% confidence interval. The 50th percentile will always be between the top and bottom 2.5 percentile portfolio values.
Question 927 mean and median returns, mode return, return distribution, arithmetic and geometric averages, continuously compounding rate
The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.
The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.
Assume that the log gross discrete returns are normally distributed and that the above estimates are true population statistics, not sample statistics, so there is no standard error in the sample mean or standard deviation estimates. Also assume that the standardised normal Z-statistic corresponding to a one-tail probability of 2.5% is exactly -1.96.
If you had a $1 million fund that replicated the ASX200 accumulation index, in how many years would the mean dollar value of your fund first be expected to lie outside the 95% confidence interval forecast?
Future prices and fund values are log-normally distributed. The mean is greater than the median, and since the median is the 50th percentile, the mean will eventually break out of the 95% confidence interval at the top. This will occur when the mean equals the 2.5 percentile dollar value. To find this time, set the mean and the 2.5 percentile value equal to each other and solve for ##t##:
###\text{MeanValue} = \text{BestTwoAndAHalfPercentileValue}### ###V_0.e^{(\mu + \sigma^2/2).t} = V_0.e^{\mu .t + \sigma.N[0.975].\sqrt{t}} ### ###(\mu + \sigma^2/2).t = \mu .t + \sigma.N[0.975].\sqrt{t} ### ###\dfrac{\sigma^2}{2}.t = \sigma.N[0.975].\sqrt{t} ### ###\dfrac{\sigma}{2}.t - N[0.975].\sqrt{t} = 0### ###\sqrt{t}.\left( \dfrac{\sigma}{2}.\sqrt{t} - N[0.975] \right)= 0### ###\dfrac{\sigma}{2}.\sqrt{t} - N[0.975] = 0### ###\begin{aligned} t &= \left( \dfrac{2.N[0.975]}{\sigma} \right) ^2 \\ &= \left( \dfrac{2 \times 1.96}{0.1692} \right) ^2 \\ &= 536.7492134 \text{ years} \\ \end{aligned}###Question 928 mean and median returns, mode return, return distribution, arithmetic and geometric averages, continuously compounding rate, no explanation
The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.
The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.
Assume that the log gross discrete returns are normally distributed and that the above estimates are true population statistics, not sample statistics, so there is no standard error in the sample mean or standard deviation estimates. Also assume that the standardised normal Z-statistic corresponding to a one-tail probability of 2.5% is exactly -1.96.
If you had a $1 million fund that replicated the ASX200 accumulation index, in how many years would the mode dollar value of your fund first be expected to lie outside the 95% confidence interval forecast?
Note that the mode of a log-normally distributed future price is: ##P_{T \text{ mode}} = P_0.e^{(\text{AALGDR} - \text{SDLGDR}^2 ).T} ##
No explanation provided.
Question 929 standard error, mean and median returns, mode return, return distribution, arithmetic and geometric averages, continuously compounding rate
The arithmetic average continuously compounded or log gross discrete return (AALGDR) on the ASX200 accumulation index over the 24 years from 31 Dec 1992 to 31 Dec 2016 is 9.49% pa.
The arithmetic standard deviation (SDLGDR) is 16.92 percentage points pa.
Assume that the data are sample statistics, not population statistics. Assume that the log gross discrete returns are normally distributed.
What is the standard error of your estimate of the sample ASX200 accumulation index arithmetic average log gross discrete return (AALGDR) over the 24 years from 1992 to 2016?
The standard error (se) of the sample average (##\bar{x}##) of a normally distributed variable is equal to the sample standard deviation of the returns (##\sigma_x##) divided by the square root of the number of observations used to calculate the sample average:
###se_\bar{x} = \dfrac{\sigma_x}{\sqrt{n}} = \dfrac{0.1692}{\sqrt{24}} = 0.03453780537###Note that the standard error is the standard deviation of the distribution of sample averages of the returns (##se_\bar{x} = \sigma_\bar{x}##), not the standard deviation of the return distribution itself (##\sigma_x##). The standard error is always less than the standard deviation of the return data (##se_\bar{x} < \sigma_x##).
Since the returns are normally distributed, then the sample average of that normally distributed variable will also be normally distributed. This is a property of the normal distribution: the sum of normally distributed variables is also normally distributed.